Relative Compaction (Rc)
It is used to indicate the degree of denseness/compactness in absolute terms as density index (ID), but it can be applied for both cohesionless & cohesive soil.
\(\mathrm{Rc}=\frac{\gamma_{d}}{\gamma_{\text {dmax }}} \times 100\)Relative Compaction is unit less.
It is defined as the ratio of the dry unit weight of soil in the existing/natural state to the dry unit weight of soil in the densest state.
\(\gamma_{d}\)= Dry unit weight of soil in existing / natural state.
\(\gamma_{dmax}\)= Dry unit weight of soil in densest state.
\(\begin{array}{l}\mathrm{Rc}=\frac{G \gamma_{w}\left(1+e_{\min }\right)}{(1+e) G_{\psi}} \times 100 \\
\mathrm{Rc}=\frac{\left(1+e_{\min }\right)}{(1+e)} \times 100
\end{array}\)
Rc= 80 + 0.2ID
In loosest state ⇒ Id=0% ⇒ Rc⇒ 80%.
In densest state ⇒ Id=100% ⇒ Rc⇒ 100%.
Calculate the value of (e)max, (e)min, (η)max, and (η)min
Packing of Uniform Sphere
- Packing of the uniform sphere is the only system, void ratio of porosity of which can be found mathematically.
- Spheres of uniform size attain stable loosest packing in a “cubical array“.
- Spheres of uniform size attain stable densest packing in “Rhombohedral array“.
1. Loosest State
Here each particle is in contact with 6 more similar particles.
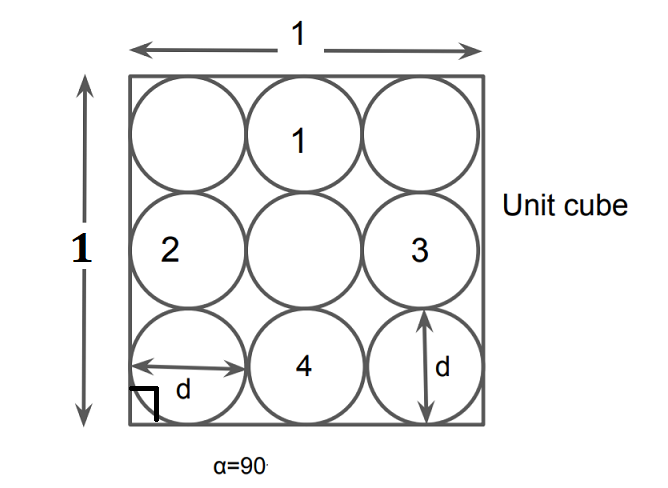
Volume of soil V=1*1*1 = 1m³.
Volume of solids in soil = (1/d)*(1/d)*(1/d) = 1/d³
The volume of one solid = πd³/6.
Total volume of solids Vs = \(\frac{\pi d^{3}}{6} \times \frac{1}{d^{3}}=\frac{\pi}{6}\)
Volume of voids Vv= V-Vs=1-(π/6)=0.476m³.
\(e_{\max }\)= Vv/Vs. = .476/(π/6) =.91
\(η_{\max }\)= \(e_{\max }\)/(1+\(e_{\max }\)) = .91/(1+.91) =.47
2. Densest State
Here each particle is in contact with 12 more similar particles.
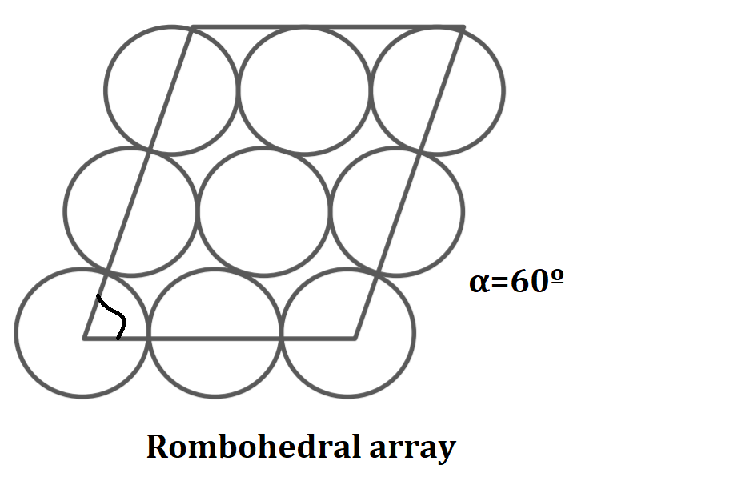
Volume of unit rhombohedral
V=\(\sqrt{1+2 \cos \alpha} \times(1-\cos \alpha)\)
Volume of soil α=60º
V=\(\sqrt{1+2 \cos60º } \times(1-\cos60º)\)=0.707m³
Volume of solids Vs = π/6.
Volume of voids Vv= V-Vs= 0.707-(π/6)=0.183m³.
\(e_{\min }\)= Vv/Vs. = .183/(π/6) =.35
\(η_{\min }\)= \(e_{\min }\)/(1+\(e_{\min }\)) = .35/(1+.35) =.26
If \(e_{\min }\),\(e_{\max }\) is not given in Question, then apply
\(e_{\min }\)=0.35,
\(e_{\min }\)=0.91.
| Subject | Soil Mechanics |
| Unit | Soil Formation & Properties of Soil |
| Topic | Relative Compaction |
| Next Topic | |
| Previous Topic | Density Index |