Unit Weight and Density | Properties of Soil | Soil Mechanics

  Unit Weight / Density  

Unit Weight is the ratio of weight to volume. It is also known as the specific weight.

\(Unit Weight=\frac{weight(Newton)}{volume(M³)}\) \(Unit Weight=\frac{N}{M³}\)

S.I Unit of Unit weight is\(\frac{N}{M³}\)

Density is the ratio of Mass to volume.

\(Density=\frac{Mass(Kg)}{volume(M³)}\) \(Density=\frac{Kg}{M³}\)

S.I Unit of Density is\(\frac{Kg}{M³}\)

---

Bulk Unit Weight (γ)/ Bulk Density(ρ)

It is the ratio of weight/mass of soil(W, M) in existing conditions to the total volume of soil mass(V). It is represented as ‘γ’ /‘ρ’.

\(\begin{array}{l}
γ=\frac{\text { Weight of soil in existing condition }}{\text { Volume of soil. }} \\
γ=\frac{W}{V} \\
\rho=\frac{\text { Mass of soil in existing condition }}{\text { Volume of soil. }} \\
\rho=\frac{M}{V} .
\end{array}\)

---

Dry Unit Weight (γ_d)/ Dry Density(ρ_d)

It is the ratio of the weight/mass of soil in dry conditions to the total volume of soil mass(V). It is represented as ‘γ_d’ /‘ρ_d’.

\(\begin{array}{l}
γ_d=\frac{\text { Weight of soil in dry condition }}{\text { Volume of soil. }} \\
γ_d=\frac{Wd}{V}=\frac{Ws}{V}\\
ρ_d=\frac{\text { Mass of soil in dry condition }}{\text { Volume of soil. }} \\
ρ_d=\frac{Md}{V}=\frac{Ms}{V}.
\end{array}\)

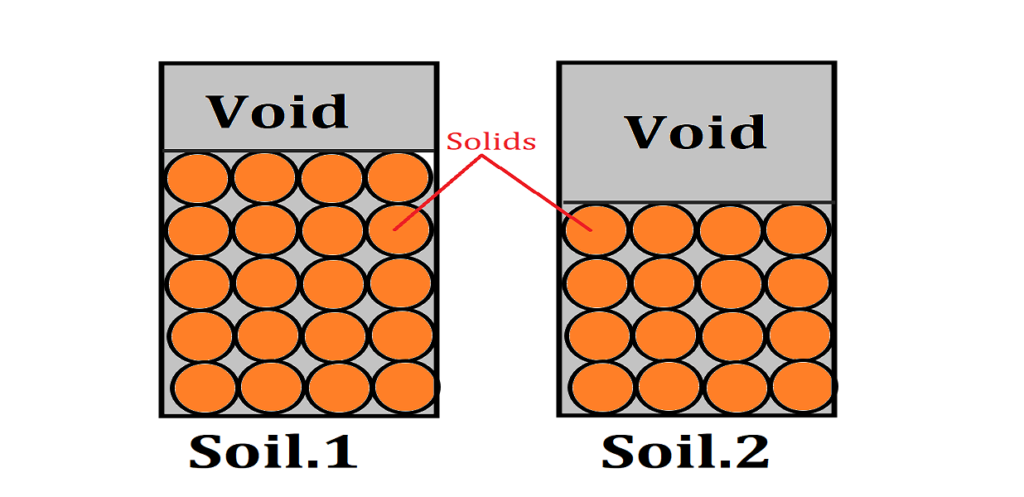
       γ_d1>γ_d2 (Ws1>Ws2)

       It can also be used to represent the denseness of soil.

       Denseness of soil  ∝  γ_d ∝ (1/e)

---

Saturated Unit Weight (γ_sat)/ Saturated Density(ρ_sat)

It is the ratio of the weight/mass of soil in Saturated conditions to the total volume of soil mass(V). It is represented as ‘γsat’ /‘ρsat’.

\(\begin{array}{l}
γsat=\frac{\text {Weight of soil in saturated condition}}{\text { Volume of soil. }} \\
γsat=\frac{Wsat}{V}\\
ρsat=\frac{\text { Mass of soil in saturated condition }}{\text { Volume of soil. }} \\
ρsat=\frac{Msat}{V}.
\end{array}\)

Note:1

• If soil is dry ⇒ s=0.
• Bulk U-W(γ)=Dry U-W (γd) or
• Bulk Density(ρ)=Dry Density(ρd).

Note:2

• • If soil is saturated⇒ s=1.
  • Bulk U-W(γ)=Saturated U-W(γsat) or
  • Bulk Density(ρ)=Saturated Density(ρsat).

---

Submerged Unit Weight (γsub)

If the soil mass is subjected below the water table level it is subjected to force of buoyancy(FB) in a vertically upward direction, the magnitude of which is equal to the weight of the water displaced by soil. which in turn reduces its weight, this reduced weight of soil is termed as Reduces/ Effective / Apparent / Buoyant /  Submerged Weight(Fb). It is represented as ‘γsub’.

\(\begin{array}{l}
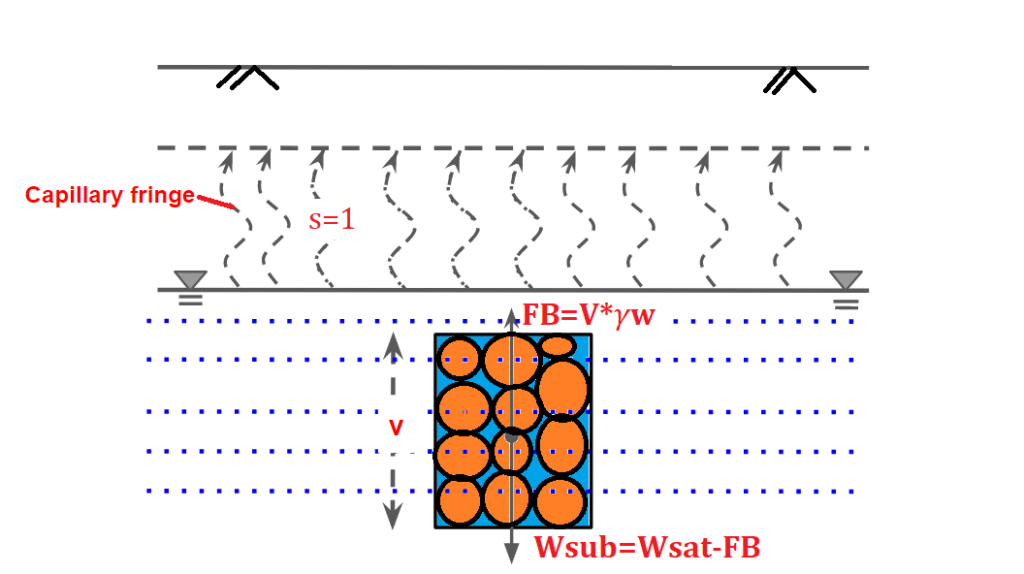
\gamma_{s u b} / \gamma^{\prime}=\frac{W s u b}{V} \\
\gamma_{s u b} / \gamma^{\prime}=\frac{W s u b-F b}{V} \\
\gamma_{s u b} / \gamma^{\prime}=\frac{W s u b-V \cdot \gamma_{w}}{V} \\
\gamma_{s u b} / \gamma^{\prime}=\frac{W s u b}{V}-\gamma_{w} \\
\gamma_{s u b} / \gamma^{\prime}=\gamma_{s a t}-\gamma_{w}
\end{array}\)

Note:

•  A soil submerged condition will always be in a saturated state, but it is not necessary a saturated soil is always submerged.
• The soil below the water table is submerged & saturated but the above water table in capillary fringe is saturated but not submerged.

---

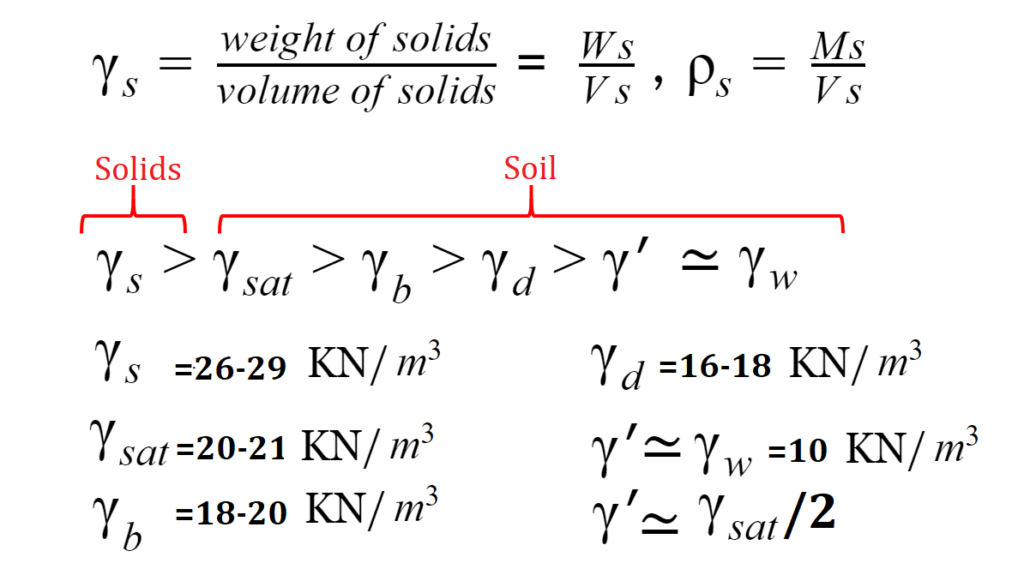
Unit Weight of Solid (γs)/ Density of Solid(ρs)

It is the ratio of weight/mass of solids(Ws/Ms) to the volume of solids mass(Vs). It is represented as ‘γs’ /‘ρs’.

\(\begin{array}{l}
\gamma_{s}=\frac{\text { weight of solids }}{\text { volume of solids }}=\frac{W s}{V s}, \rho_{s}=\frac{M s}{V s} \\
\gamma_{s}>\gamma_{s a t}>\gamma_{b}>\gamma_{d}>\gamma^{\prime} \simeq \gamma_{w}
\end{array}\)

\(γ_w\)= U-W of water= 9.81kn/m³.

---

Subject	Soil Mechanics
Unit	Soil Formation & Properties of Soil
Topic	Unit Weight and Density
Next Topic	Specific Gravity
Previous Topic	Degree of Saturation, Air Content, Percentage Air Voids

---

FOR MORE INFORMATION ABOUT SOIL MECHANICS